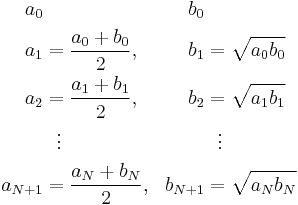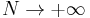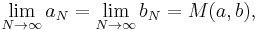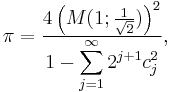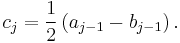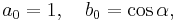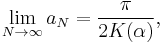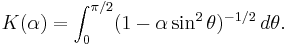AGM method

In mathematics, the AGM method (for arithmetic-geometric mean) makes it possible to construct fast algorithms for calculation of exponential and trigonometric functions, and some mathematical constants and in particular, to quickly compute \pi.

Gauss noticed [1][2] that the sequences

\begin{align} a_0 & & b_0 \\ a_1 & = \frac{a_0%2Bb_0}{2}, & b_1 & = \sqrt{a_0 b_0} \\ a_2 & = \frac{a_1%2Bb_1}{2}, & b_2 & = \sqrt{a_1 b_1} \\ & {}\ \ \vdots & & {}\ \ \vdots \\ a_{N%2B1} & = \frac{a_N %2B b_N}{2}, & b_{N%2B1} & = \sqrt{a_N b_N} \end{align}

have for

N\to %2B\infty \,

the same limit:

\lim_{N\to\infty}a_N = \lim_{N\to\infty}b_N = M(a,b), \,

the arithmetic-geometric mean.

It is possible to use this fact to construct fast algorithms for calculation of elementary transcendental functions and some classical constants and in particular, to quickly calculate the constant \pi .

For example, according to the Gauss–Salamin formula:[3]

\pi = \frac{4 \left( M(1; \frac{1}{\sqrt{2}}) \right)^2} {\displaystyle 1 - \sum_{j=1}^\infty 2^{j%2B1} c_j^2} ,

where

c_j = \frac 12\left(a_{j-1}-b_{j-1}\right).

At the same time, if we take

a_0 = 1, \quad b_0 = \cos\alpha,

then

\lim_{N\to\infty}a_N = \frac{\pi}{2K(\alpha)},

where K(α) is a complete elliptic integral

K(\alpha) = \int_0^{\pi/2}(1 - \alpha \sin^2\theta)^{-1/2} \, d\theta .

Using this property of the AGM and also the ascending transformations of Landen,[4] Richard Brent[5] suggested the first AGM algorithms for fast evaluation of elementary transcendental functions (ex, cos x, sin x). Later many authors have been going on to study and use the AGM algorithms, see, for example, the book "Pi and the AGM" by Jonathan and Peter Borwein.[6]

See also

References

  1. ^ B. C. Carlson (1971). "Algorithms involving arithmetic and geometric means". Amer. Math. Monthly 78: 496–505. doi:10.2307/2317754. MR0283246. 
  2. ^ B. C. Carlson (1972). "An algorithm for computing logarithms and arctangents". Math.Comp. 26 (118): 543–549. doi:10.2307/2005182. MR0307438. 
  3. ^ E. Salamin (1976). "Computation of \pi using arithmetic-geometric mean". Math. Comp. 30 (135): 565–570. doi:10.2307/2005327. MR0404124. 
  4. ^ J. Landen (1775). "An investigation of a general theorem for finding the length of any arc of any conic hyperbola, by means of two elliptic arcs, with some other new and useful theorems deduced therefrom". Philos. Trans. Royal Soc. London 65: 283–289. 
  5. ^ R.P. Brent (1976). "Fast Multiple-Precision Evaluation of Elementary Functions". J. Assoc. Comput. Mach. 23 (2): 242–251. doi:10.1145/321941.321944. MR0395314. 
  6. ^ J.M. Borwein, P.B. Borwein (1987). Pi and the AGM. New York: Wiley. ISBN 0-471-83138-7. MR0877728.